Reversi --
History, Curiosities, and Variants
by Michael Keller
Special thanks to:
Gunnar Anderssen, who permitted me the use of his excellent program Zebra
(developed by Anderssen and Lars Ivansson) for making many of the
diagrams here. Zebra is a freeware program which runs under
Windows (there is also a version for Android). It is a
strong playing program with many features.
Graham Brightwell, editor of the British Othello Federation Newsletter, who allowed me to republish some constructed games.
Aubrey de Grey, who constructed many of the games in this article and permitted me to publish them in WGR.
One of the most popular abstract games with simple rules is Reversi. It was published in the 1880's as a proprietary game,
played on the 8x8 board still familiar today, invented by Lewis
Waterman. A rival inventor, John W. Mollett, claimed to have
invented the game of Annexation in 1870, which had similar rules (in
particular, the same type of reversal capture), but was played on a
cross-shaped board. Each man claimed the other was a
fraud. If Mollett's claim is true, he apparently had
the capture idea before Waterman (Waterman may have taken the idea
from Mollett or arrived at it independently). Waterman's claim is supported by newspaper
accounts from the time, and a handbook devoted to the game. I
have a handbook of games published in 1890 (obtained from Philip Cohen)
which includes a 55-page section on Reversi, with complete rules and
quite a detailed discussion of strategy. Many
accounts of the history of Reversi have been published, but there is
much confusion in exactly what dates various events occurred. Since the
modern game of Reversi uses
Waterman's board and name, I would say that Lewis Waterman deserves the
title of inventor. I named my own Reversi program Waterman
in his honor.

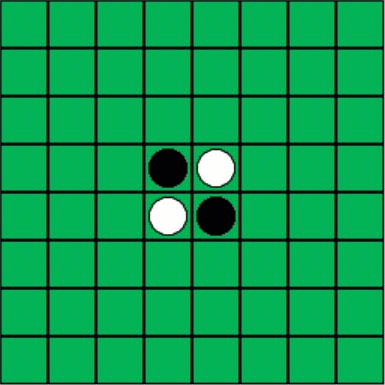
The two essentially different starting positions (orthogonal and oblique) in Reversi
Reversi is played by two players on an 8x8 board (usually uncheckered),
with 64 two-sided pieces with different colors on each
side. For simplicity, we will assume the pieces are black
and white, and the players are designated Black and White (unlike
chess, Black moves first). Players play alternately,
placing a single piece of their color on each turn. The
first four pieces must be played in the center four squares, leading to
one of the two configurations shown above (all other configurations are
reflections or rotations of one of these two). On each successive
play, each player must flip one or more opposing pieces by a form of custodian capture
(but reversing the color of the pieces, rather than removing
them). That is, each new piece placed must be adjacent to
at least one opposing piece which is in line (orthogonally or
diagonally) with a friendly piece, with no gaps. All
opposing pieces between the two friendly pieces must be flipped over to
the moving player's color. A newly played piece can form
custodian captures along multiple lines: the opposing pieces along
every line are flipped.
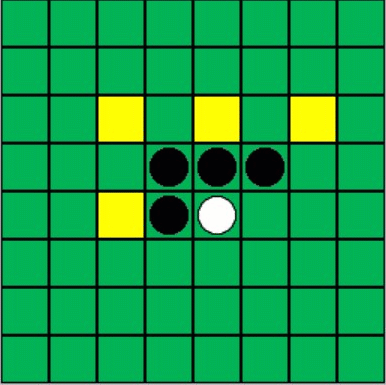

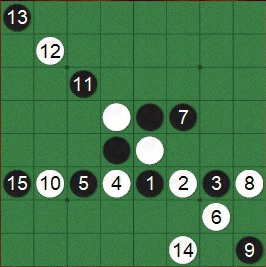
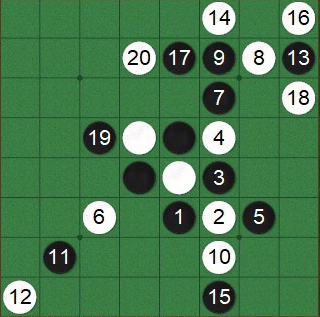
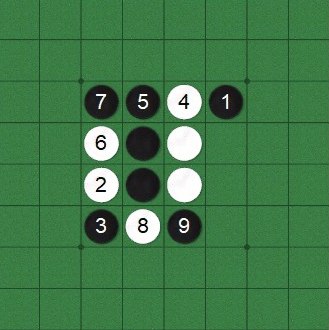
Examples: from the orthogonal start,
Black played f5 and flipped e5 (between d5 and f5); White can play in
any of the yellow squares. White played c4 and flipped
d4. Black then flipped pieces along two lines by playing d3
(d5 allows d4 to flip; f5 allows e4 to flip).
A player who cannot move passes his turn; the opponent then plays again
until the player who passed has an available move. Play
continues until neither player can move (usually when the board is
completely filled, or all of the pieces are of one color, though double
stalemates are possible). The player with more pieces
of her color wins (ties are also possible).
I originally learned the game in my childhood through
accounts in
various game compendia (I still have a set of rubberized black and red
pieces from World Wide Games), and played postally in the Knights of the
Square Table in the 1970's. We played under one rule
different from Waterman's rules: in the original rules each player had
32 pieces, and if your opponent had to pass a move you would run out of
pieces first, and the opponent could play extra moves at the end. This
is an undesirable rule, since it makes having to pass possibly
advantageous at the end of the game. We used a rule which is nearly universal now: there
is a common pool of 64 pieces, and if you are forced to pass, you
simply skip your turn until a play by the opponent allows you to move
again. Martin Gardner discussed the game in his
Mathematical Games column in the April 1960 issue of Scientific
American.
Two readers sent in wipeout wins of 11-0, starting from the orthogonal Reversi position; one of these is shown below. The updated column was reprinted in 1966 in the collection New
Mathematical Diversions from
Scientific American: Chapter 6, Board Games).
The game achieved great popularity when a Japanese inventor,
Goro
Hasegawa, reintroduced the game as Othello. This was trademarked
and published commercially by the Tsukuda Original company in Japan in
1973. With help from James R. Becker, Hasegawa formed the Japanese Othello Association
(still active), wrote a book, and promoted the game, which spread all
over the world. Although Hasegawa claimed to have invented
the game in 1971, Othello is actually identical to the game of Reversi
as I learned it, except that Othello starts from a fixed opening
position, while in Reversi the players place their first two pieces
alternately in the center, which can lead to two essentially different opening
positions. (These positions can converge with as few as eight
pieces on the board, as we will see momentarily). The US Othello
Association was formed in 1978, and published an excellent magazine, Othello Quarterly, from 1979 to 2005. The British Othello Federation also publishes a bulletin.
The notation used by most Othello
organizations, following Japanese
practice, has a1 at the upper left corner -- an unfortunate
choice, in my view. WGR advocates the consistent
use of standard algebraic notation in all board games where practical,
even where this may push aside traditional
notations such as checkers (where traditionally the squares are simply
numbered 1 to 32). In this article we will mostly
show games in graphical format, with the moves numbered (some games may
be rotated or flipped).
Curiosities in Reversi and Othello
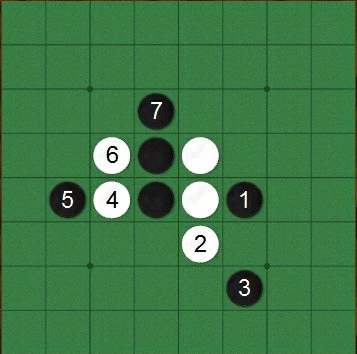
In the July 1993 British Othello Newsletter (Forty Billion Nodes Under The Tree), Sid Cox's article "Syncopated Cerebrations" asks how early can an Othello game and a Reversi game
(from the orthogonal starting position) reach the same
position? The answer is four
moves (shown below); this is a well-known result, and there is at least one other four-move solution):
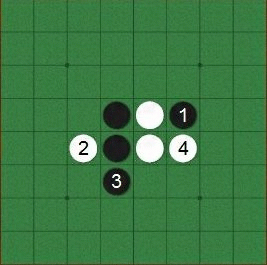
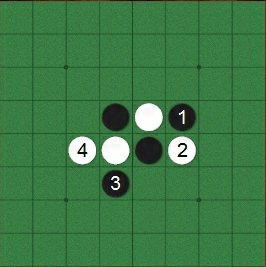
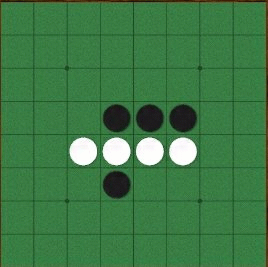
A
question posed by David Haigh (Othello Quarterly Vol. 3, Num. 2, Fall 1981, pages 30-31, letter to the editor):
what is the earliest possible pass
after which play continues (i.e. it does not result in an immediate
wipeout or stalemate)? I found an 8 move solution, which
appeared in WGR13 (this is also a well-known result); Black must pass after
White's 8th move, but the
game will continue, although White should win easily by forcing Black
to g7 and eventually taking the corner at h8. It is later
possible to reach a position where Black has 16 straight moves with
either a pass or a forced move, ending in a 44-0 wipeout (de Grey
constructed a game ending with 17 consecutive forced moves or passes,
ending in a 64-0 wipeout).
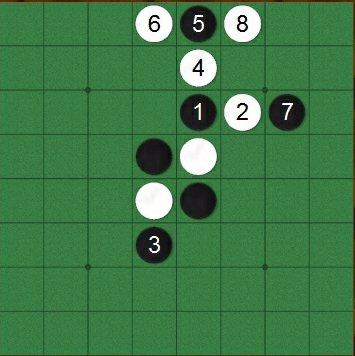
One of the top British Othello players, Aubrey de Grey,
constructed the shortest known game of Othello not ending in a wipeout
(Black winning 15-4); this appeared on the cover of the January 1993
British Othello Federation Newsletter (The Killer Bees).
Editor
Graham Brightwell then challenged readers to construct the shortest
possible game ending in a draw (naturally the final position must be a
double stalemate). In May 1993 The British Othello Federation
published the first British Othello Bulletin, a 4-page supplement to
its 48-page Newsletter. The following game
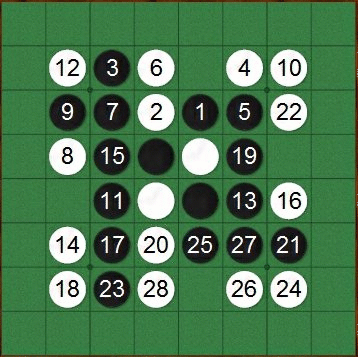
appeared on page 1 without comment: 1 e6 2f6 3c4 4d6 5f7 6c3 7c6 8c7
9g6 10h6 11c8 12g8 13c2 14b2 15a2 16c1 17g7 18c5 19b3 20b6 21a6 22h8
23e3 24a3 25f4 26a1. This game, again from de Grey, ends up
in a 15-15 tie -- Graham Brightwell commented to me that he had never
even heard of a 31-31 tie in actual play! [Colin Springer, in a letter to the editor published in Othello Quarterly, Vol. 18, Num. 4, Winter
1996-1997, page 2, gave the transcript of a 31-31 tie which
occurred in a game between Eclipse, a computer program co-written by Springer, and another program,
Brutus.] Aubrey de Grey later constructed an even shorter drawn game, ending 12-12. If Black plays c5 on move 17
instead of move 19, White can only play at h6, and the game ends in a
12-10 win for Black. This is the smallest
known winning disc count in an Othello game, even beating out the 13-0
wipeouts (see the Bibliography for more on wipeouts).
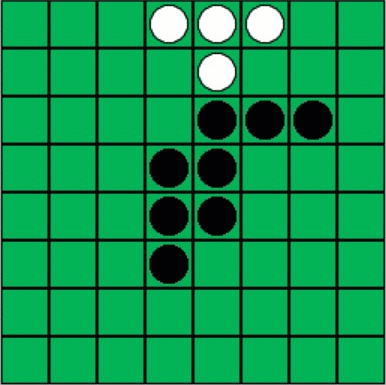
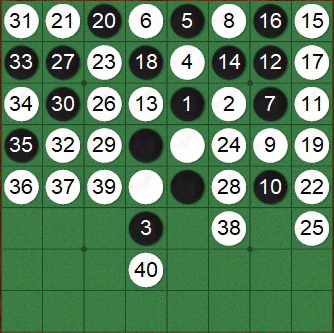
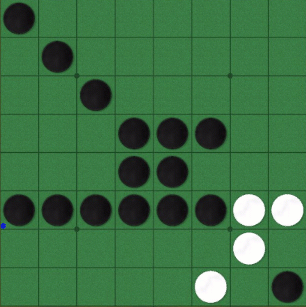
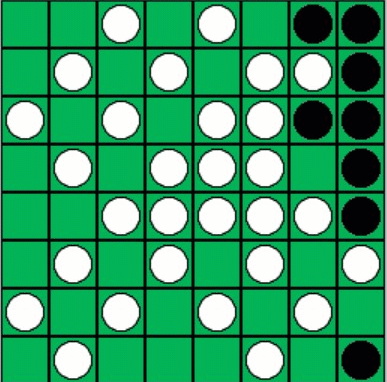
Another of Aubrey de Grey's ingenious constructed games is a
64-0 wipeout win by Black; after White's move 33 the position is
shown (below, center). Black then makes 12
consecutive plays (White must pass 11 times) before White can play
again (below right, White about to make move 46). Black then finishes the game with 14
straight plays (with 13 passes by White). Including a pass after
move 31, White makes 25 passes in all, making only 17 moves in the
entire game.

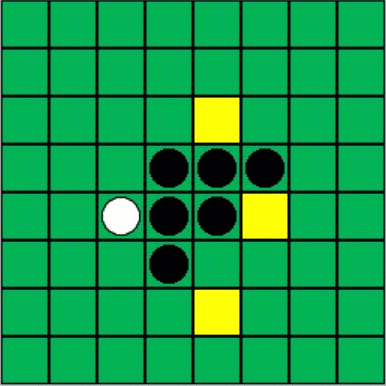
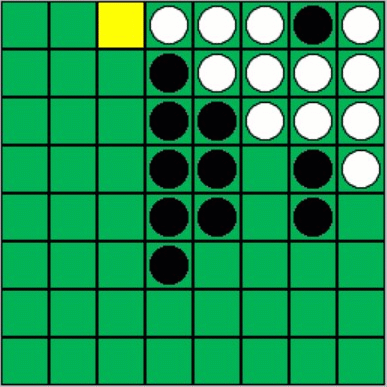
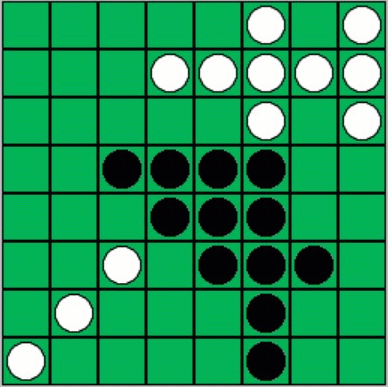
Another construction by de Grey is a position,
with Black to play, in which Black can move to every one of the 32
empty squares. The moves to
reach this position are shown below left, and the resulting position
(possible squares to which Black can move are highlighted in yellow) is
shown below, right:
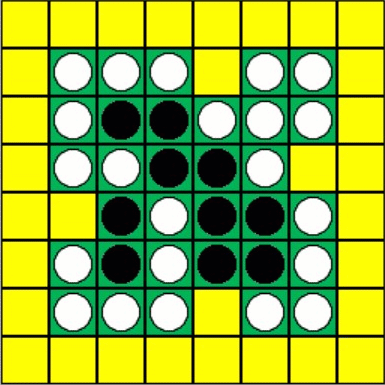
Another game by de
Grey is a 16-1 win for Black, the shortest known
win not ending in a wipeout: this was based on an actual game between
Denis Rouillon and Laurent Pelissier in the 1989 French national
championship, which ended in a 21-1 win by White.
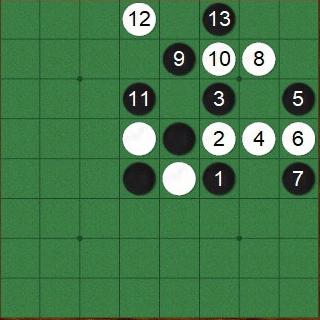
Only one flip per turn
Here is a problem I thought of recently: what is the longest
possible sequence of moves, from the start of a
game (using either starting position), in which only one disc is
flipped on each move?
My best effort was 41 moves. I sent the
problem to Donald Knuth, who quickly responded with a near-perfect 59
move sequence, as well as a perfect 6x6 solution.

Tracy Cobbs, a game player and writer, who was a top-30 tournament player in Othello for a while, created the following
palindrome, imagined to be
spoken by one of the two rival inventors of Reversi in the 1880's :
"I made Reversi. 'Tis revered -- am I?"
Variants of Reversi
Some sources have incorrect rules:
(1) The game ends as soon as one player cannot play.
(2) Flips can be made along only one line per turn (Ravensburger gave this as a variant for children)
The most obvious variant of Reversi is to play on a larger board.
It seems reasonable that 10x10 Reversi should play
well; 10x10 boards made for checkers and even chess are widely
available. Smaller boards (4x4 and 6x6) have been completely solved by
computer (4x4 is a very easy win for White going second). In the July 1993 BOF Newsletter (Forty Billion Nodes Under The Tree), Joel
Feinstein presented analysis by his Othello program MODOT, showing that
White (playing second) wins in 6x6 Othello, with best play by both sides
("Amenor Wins World 6x6 Championships", pp. 6-8). Computers were already playing better than the best human players as early as 1997: Logistello, a powerful Othello program written by Michael Buro, won a
six-game exhibition match August 4-7, 1997, against World Champion Takeshi Murakami, with a surprising 6-0
score. The match appeared to confirm that the strongest Othello
programs are stronger than any human players. In November 2023, Hiroki Takizawa published a paper, Othello Is Solved,
announcing that 8x8 Othello is a 32-32 draw with perfect play by both
players. This is known as weakly solving: the program,
Takizawa's modification of an algorithm called Edax, can play optimally
from the starting position.
Another obvious variant for 8x8 Reversi is to allow the first play to
be made anywhere, but the first four plays must still form a 2x2 square
somewhere.
Misere Reversi, in which
the object is to end up with the smallest number of discs, has also been
studied. This is also known as anti-reversi.
Reversi can easily be played on a hexagonal grid, with the board either
a hexagon or parallelogram. A hexagon five on a side gives
a board of 61 cells and a game comparable in length to 8x8
Reversi. Christian Freeling invented a variant, MacBeth,
on a hexagonal board six on a side, but with 19 symmetrically placed
holes, so that every playable cell lies along only two lines.
Sid Cox's article "Syncopated Cerebrations"
asked how Othello can best be handicapped: possibly by allowing the weaker player
one or more 'passes' which allow him to skip a move at any time.
Commercial variants
Domain - invented by Claude Duvernay, published by Parker Brothers,
1983, $13
Domain is an interesting and original game of the Reversi
family. It is played on a 9x9 board, using pieces
with eight different symmetric polyomino shapes, ranging in
size from a bar-shaped piece which covers two squares, to a
cross-shaped piece which covers five squares (an X pentomino). Each
piece has two sides, blue and white. The two players (one playing blue,
the other white) alternate placing pieces on the board with their color
face up. After placing a piece, the player flips every orthogonally adjacent piece of the opposite color to his
own color. The object is to have a larger area
covered by your color when no more pieces can be played. Three levels
of play are provided; in the expert level, all adjacent pieces,
regardless of color, are flipped after each piece is played. The
physical components are up to Parker Brothers' usual high
standards. The board and pieces are sturdy plastic, and the
pieces have pegs which fit into the holes on the board, keeping
them from shifting out of position. Experience in Reversi may be
somewhat helpful, but the Domain player will have to work out most of his strategies himself. One comment to Parker
Brothers: the inventor of a game deserves to be credited.
Ataxx - invented by Dave Crummack and Craig Galley, 1988, first released by Leland, 1990, arcade
Ataxx is a board game which began as a computer game, originally called Infection. The first commercial version was the arcade
game Ataxx in 1990. It later appeared on
both Nintendo and IBM systems as Spot; Tracy Cobbs introduced me to the
game
through the Nintendo version.
There are now many computer versions, including a hexagonal version
unimaginatively called Hexxagōn. The game itself is a simple and
elegant abstract board game
with some similarities to Reversi and Domain. The usual
board is
7x7; 49 Reversi-style pieces (e.g. black on one side and white on the
other)
are
needed. Initially the player going first has pieces at a7
and g1; the second
player has pieces at a1 and g7. Players alternate moving one
piece per
turn. (Many computer versions block out squares on the
board in various patterns, but we will consider the plain 7x7
game. A very detailed set of pages on Ataxx (in French and English) are on Alain Beyrand's Pressibus site (he also has a Reversi site).
There are two kinds of moves :
(1) A piece adjacent to an empty square (orthogonally or diagonally,
like a king in chess) can create a new piece in the
empty square. E.g. if there is a piece at c3, and d4 is vacant, a
new piece can be created in d4. Since it doesn't really
matter which adjacent square was involved, we notate this move with the landing
square only : e.g. d4 indicates that a piece was created on d4 from
one of the adjacent squares occupied by a friendly piece.
(2) A piece can jump into an empty square two squares distant
(orthogonally, diagonally, or by a knight's move -- this is
like the fairy chess piece called the squirrel) -- intervening
squares may be leaped whether empty or occupied by friendly or enemy
pieces. No new piece is created. A piece at c3
may jump to a1, a2, a3, a4, a5, b5, c5, d5, e5, e4, e3, e2, e1, d1, c1,or b1 -- in any case
c3 will be vacant after the move. We notate such a move (e.g.)
c3b5.
After a move, enemy pieces adjacent
(kingwise) to the new piece's square in case (1) or the landing square
in case (2) are flipped over as in Reversi. The number of
pieces flipped over as a
result of each move is noted in parentheses. The game ends
when the board
is full; the winner is the player with a larger number of pieces (ties
are impossible because of the odd board size). Here
is a
sample game Tracy Cobbs (White) and I (Black) played by post and e-mail, 1994-1995:
1
b7 b2 2 f1 c3 3 g2 f6 4 e1 d2(1) 5 e2(2) b2d1(3) 6 f2(2) e3(2) 7 f3(3)
d3(2) 8 f3d4(3) e2e4(3) 9 e2(4) f3(4) 10 c3e5(3) g7f5(3) 11 d4f4(5)
f6d4(4) 12 b7d5(3) d3c5(2) 13 d3(3) c4(2) 14 c3(3) a1c2(4) 15 a7c6(2)
b4(2) 16 b5(3) b3(2) 17 g3(2) b4d6(4) 18 e6(3) b4(1) 19 d6b6(3) b4d6(5)
20 f6(2) b4(1) 21 d7(2) d5e7(4) 22 d5(4) f6g4(4) 23 b6a4(3)
c3a5(3) 24 c3(4) c1(2) 25 f6(2) d7f7(3) 26
b2(2) a3(3) 27 c1a2(3) c1(2) 28 b1(3)
b6(2) 29 c7(2) d7(3) 30 g6(2) g5(3) 31
a6(2) Resigns
Position
7 - - X X X O -
6 O O X X X X X
5 O O X O O X X
4 X X O O O X X
3 O O O O O X X
2 O O O X O O O
1 - O O X O O O
a b c d e f g
Bibliography
Several of the best strategy guides in English are available in
electronic format (PDF).
Ball,
John A. and Parker, John R. -- The Key To Othello, 1981
One of the earliest books on Othello strategy, written by two British
players. I have never seen a copy; it is extremely rare.
I cannot find it in any of the usual online catalogs: Library of
Congress, Bookfinder, or Worldcat. (Othello is a terrible
name for a board game for search purposes, as the vast majority of
references are to Shakespeare's play.)
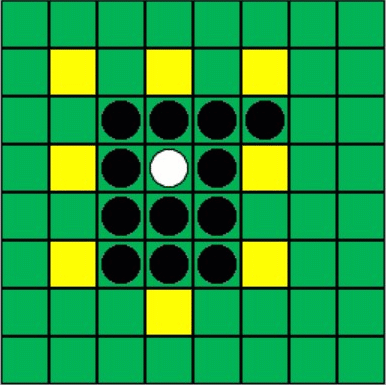
Berkeley -- The Handbook of Games, Volume I: Table Games, 1890, George Bell and
Sons
Reversi is covered on pages 1-57 of the section Reversi
and Go-Bang; there is also a description of the multiplayer variant
Royal Reversi on pages 58-61. Berkeley was one of the best 19th
century writers on games, and this is a surprisingly good piece on work
on a game only a few years old. It includes 15 pages on
openings (though it relies on what is now known as the Sweet Sixteen
principle, which is of lesser importance in modern opening
theory). Page 29-30 (Fool's Mate) shows an opening mistake
which puts leaves White with one piece, completely surrounded, so that
any of 8 possible moves is answered by a 15-0 wipeout (diagram above).
Cady, Alice Howard -- Reversi, c.1896, American Sports Publishing Company
Monthly installment of Spalding's Home Library. I have
only seen a listing for this in advertisements in other books by
Cady. It is listed as number 15, so possibly it was
published in September 1896 (Hearts, number 14, was August
1986). Likely about 40 pages disregarding ads.
Dawson, Lawrence -- Hoyle's Games Modernized, 1950, Routledge & Kegan Paul, 480 pp., hardback
Reversi is covered on pages 447-449, with the modern rules (a
player who cannot move passes until he can move again, there is no
32-move limit). Dawson gives a brief description of good
and bad squares, and says of the placement of the third piece: "...it
is generally considered to be bad play on the part of the first player
if he places his diagonally." Internet Archive has a
copy of the 1994 edition (ISBN 1-85326-316-8) available for borrowing.
Fang, Randy -- Othello: From Beginner To Master, 2003, Fang, 64 pages
Available online.
Hasegawa, Goro, translated by Maxine Brady -- How To Win At Othello, 1977, Harvest/HBJ, 122 pp., paperback, ISBN 0-15-642215-8, $2.95
A notoriously bad book, difficult to understand and full of
mistakes. English edition of the 1974 Japanese
original. A detailed assessment is in the Spring 1980 Othello Quarterly. Out of print, but not hard to find used.
Hewlett, Clarence -- Perfect Play Sequences for 315 Normalized Positions with 20 Empty Squares, 1990, Hewlett, 26 pp., $3
A set of analyses which derived using Hewlett's customized
computer hardware for analyzing Othello endgames. Each of
the 315 positions is encoded and sorted alphabetically, and accompanied
by a diagram, moves showing best play for both sides until the end of
the game, and a final score. This should be useful for both
human and computer endgame study.
Landau, Ted -- Othello: Brief & Basic, 1985, 1987, U.S. Othello Assocation, 63 pp., paperback, $6.00
The first comprehensive guide to Othello strategy, written by the editor of Othello Quarterly. The 1990 second printing of the 1987 revised edition is available on Ted Landau's website.
Landau, Ted, ed. -- Othello Quarterly, 1979-2005, U.S.
Othello Association, quarterly, 32 pp.
My collection runs from Spring 1979 (Vol. 1 Num. 1) through at least Winter 2002 (Vol. 24 Num.4), but with some gaps.
Lazard, Emmanuel
An authorized online version is available at Gunnar Anderssen's website.
Levy, David - Computer Gamesmanship, 1983, Simon and
Schuster
Early guide to programming Reversi.
Rose, Brian -- Othello: A Minute to Learn...A Lifetime To Master, 2005, Rose, 162 pp., paperback
The most up-to-date strategy guide, available for free download in PDF format.
Stepanov, Oleg -- 100 Problems For Beginners, 1989, Leningrad, pamphlet, 21 pp., $3
A collection of midgame and endgame problems in diagram form, with solutions.
Curiosities in Othello and Reversi
Gardner, Martin -- Martin Gardner's New Mathematical Diversions from
Scientific American, Simon & Schuster, 1966
Chapter 6, Board Games: a reprint and update of Gardner's April
1960 Mathematical Games column in Scientific American. Two readers
sent in first-player Reversi wipeout wins of 11-0 (pages 80-81).
Haigh, David -- Othello Quarterly Vol. 3, Num. 3, Winter 1981/1982,
page 28
Letter to the editor, stating that there are only four 11-0 wins in
Reversi (7 d2 can be played in the solution shown, and/or moves 8/9 can
be swapped with 10/11).
Haigh also programmed a computer to look for the shortest possible
games starting with the Othello opening position
(equivalent to 1 d5 2 e5 3 e4 4 d4). He
found 57 different games ending in
13-0 wipeouts for Black (confirming published analysis in 1975 by a
Japanese player, Manabu Maruo, who
also found 89 games ending in 14-0 wipeouts for
White.) The most interesting is: 5 e6 6 f4 7 e3 8 f6 9 g5 10
d6 11
e7 12 f5 13 c5, with the pieces ending in a perfect diamond. Maruo
found a similar game winning 14-0
for White: 5 d3 6 c5 7 e6 8 d2 9 c4 10 f5 11
c6 12 b5 13 d6 14 d7
Springer, Colin -- Othello Quarterly, Vol. 18, Num. 4, Winter
1996-1997, page 2
Letter to the editor, giving the transcript of a 31-31 tie which
occurred in a recent game between Eclipse, a computer program co-written by Springer, and another program,
Brutus.
Much of the material herein appeared in various issues of WGR; in
particular the section Curiosities in Reversi and Othello is an update
of articles in WGR12 (p.7) and WGR13 (p. 15).
This article is
copyright ©2024 by Michael Keller. All rights reserved.
This file was revised on July 17, 2024.

























