Pyramid Puzzlements

This is a legacy article to avoid broken links. A revised version is part of the Pyramid article here. The specific section with this article is here.

Tutenkhamen's Curse, or Pyramid Puzzlements

Why Pyramid Must Have The Top of The Stock Available

In 1990, Microsoft released the second of its Windows Entertainment Packs, which contained two card solitaires. The first of these was FreeCell, which became a worldwide phenomenon. The second was a variant of Pyramid called Tut's Tomb, which did not.

Tut's Tomb features (in one variant) a three-at-a-time deal from the stock to a wastepile (lifted from the game Demon). [The alternate version, where the stock is dealt one card at a time without a redeal, is pretty well hopeless.]. The top of the stock is not available, and the top of the waste can only be matched with an exposed card from the pyramid. (The same mistake can be found in a number of other computer versions of Pyramid.) The object of the game is to clear the pyramid of 28 cards only, and while the game is playable (i.e. it can be won occasionally), it is much less interesting than most Pyramid variants where all 52 cards must be discarded -- the skill in playing Pyramid lies in knowing what matches to make and which to pass up, and when to match stock-to-waste and when to match within the pyramid. Tut's Tomb allows a card in the pyramid to be matched with a card directly underneath it if that card is not covered by any other card (e.g. in the second diagram, the two of clubs and jack of hearts could be removed together after the four of spades had been removed; removing the queen of spades would allow the eight of clubs and five of hearts to be matched). This rule helps only a little.

In some versions of the game, the object is to discard all 52 cards. What does this mean? Since every card from the stock of 24 (except kings) must be matched with a card from the pyramid of 28, it means that if all four kings are on the pyramid, there can be no pyramid-to-pyramid matches. Each king in the stock leaves room for one pyramid-to-pyramid match. Since most pyramids require a few matches within the pyramid to unblock troublesome ranks, this makes breaking down the pyramid extremely difficult. [Some computer versions of Pyramid make a different mistake, automatically discarding both cards (if they are complementary) when the top of the stock is placed on the waste to make room for a new stock card. Frequently one needs such a pair to break up a pair in the pyramid where one is covering the other. I don't know how to quantify how much of a difference this makes.]

But the situation is worse than that. Since stock-to-waste matches are not possible, the stock cannot contain more than four of any pair of complementary ranks (e.g. if there are 2 fives and 3 eights in the stock, there will be no way to discard the last one of each). Some of the pairs may have less than four, the combined shortfall being the same as the number of kings in the stock. What are the odds of the stock containing a suitable combination of ranks? I did a combinatorial calculation (some mathematically inclined reader may care to confirm this) showing that 5.547 percent of the deals are acceptable -- a computer simulation of two million deals also gave a figure slightly above 5.5 percent. This means that if you cannot pair up within the stock, you can win at Pyramid 5-1/2 percent of the time if any card from the stock can be matched with any complementary card from the pyramid regardless of its location. Since the pyramid's shape makes cards available only gradually, and the three-at-a-time deal makes matters worse, I can't imagine the win rate being more than about half a percent, and perhaps much less. And that win rate assumes that the player knows that the number of pyramid-to-pyramid matches is limited by the number of kings in the stock.

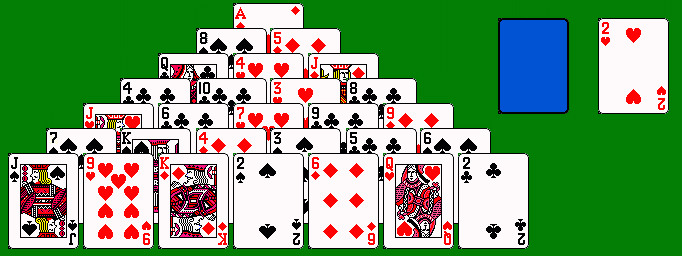
Pyrimpos.gif (15764 bytes)

An impossible deal: If the top of the stock is not available to be matched with the top of the waste, this Pyramid deal (screen shot from the Solitaire Laboratory program) cannot be won. There are two queens and three aces in the stock, and when three queen-ace matches have been made between the stock and pyramid, there will be one queen and one ace left in the stock and no way to discard them.

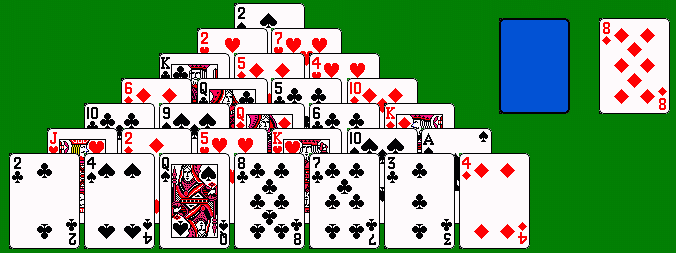
Pyrpos.gif (14805 bytes)

A possible deal: There is one king in the stock, thus 23 non-kings in the stock and 25 in the pyramid. One pyramid-to-pyramid match will be possible, which must be a jack-two match, specifically the jack of hearts and two of diamonds (the only two which is neither covers nor is covered by the jack). Such deals occur infrequently, and most have three or four kings in the stock. All other matches must be stock-to-pyramid.

When King Tut (modeled after the original Tut's Tomb) first appeared in Pretty Good Solitaire in 2001, the rules specified that all 52 cards must be discarded, and yet the Top Scores page listed 42 wins out of 408 tries. I suspect that some people were submitting doctored stats (interestingly, neither of the players who at that time had played enough games to be listed individually had won even once.) BVS Solitaire Collection's top score pages do not appear to have the same game, so I cannot compare its stats. King Tut's rules were later modified to require only the pyramid to be cleared. I still don't consider it to be a good game (much better is the three-deal Par Pyramid, with one card at a time dealt and the top of the stock available); I consider the three-at-a-time deal to be an overused mechanism which doesn't work well in most games, including Pyramid.

Does anyone out there have a fully winnable deal of King Tut (i.e. a deal number which can definitely be won by discarding all 52 cards)? I'm curious to know if there is anything wrong with my analysis.